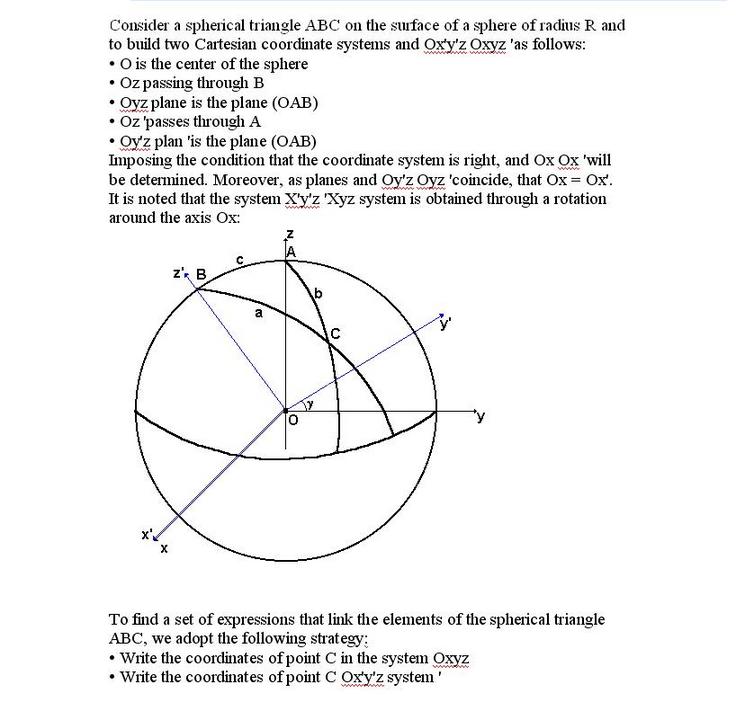
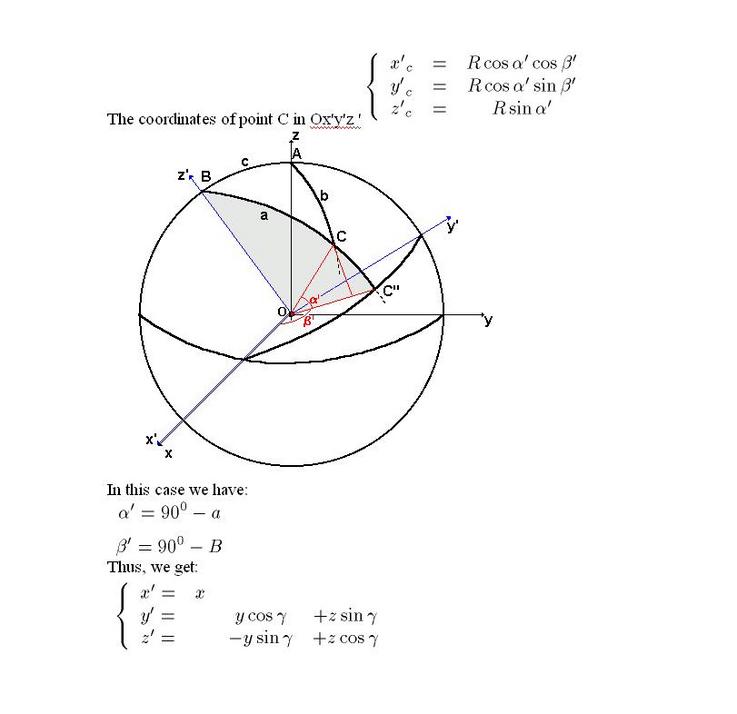
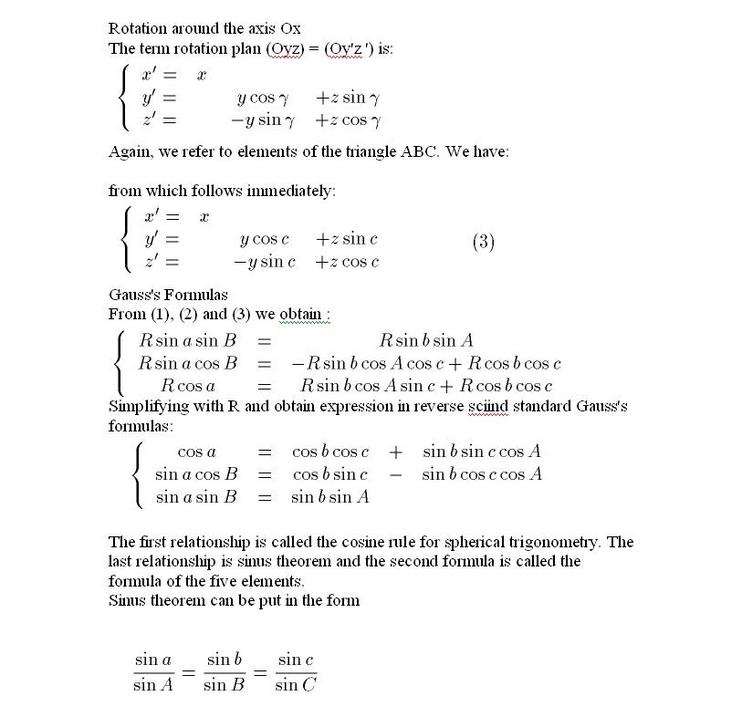
Toricelli's Points ( By Daniel Tica ) :
Torricelli-FARM'S POINT
Torricelli's 1.Teorema. Ferman's Theorem
Theorem 1 (Torricelli). Consider a triangle ABC in common with all angles strictly less than 120 ° SIP is being built in outer sides of the triangle equilateral triangles ABC, ACB, BCA. Circumscribed circles of these triangles have
Theorem 1 (Torricelli). Consider a triangle ABC in common.
Proof:
T be the point of intersection of the circles of triangles ABC and ACB. It proves that the quadrilaterals BTCA is writable. This is seen as quadrilaterals C BTA and TB were involved recordable BTAΞATC € 120 ° angles from which it follows that the quadrilateral BTCA is writable.
Note.
In this way, and demonstrated that there is a single point in the plane T with the property that ATB, ATC, BTC € 120 °, since T must belong to the three circles considered simultaneously. Point T is called Torricelli point for triunghil ABC's.
Theorem 2. Consider triangle ABC with all angles strictly less than 120 ° and equilateral triangles AB C, AC B BC up outside that in the previous theorem. Then:
a) AA, BB, CC are concurrent.
b) AT + BT + CT = DC = AA = BB
Proof. T be the point of competing circles of the problem before. It demonstrates that A, T, A are collinear, hence will result as AA, BB, CC are concurrent in T.
Uniting T to obtain: A TC, CBA, € € 60 ° and 120 ° ATC, ATA € 180 ° angle so.
For point b) proceed as follows: AA = AT + TA.
Ptolemy's theorem applies to recordable BTCA apentru quadrilateral (which is an equilateral triangle BCA) follows BT + TC = TA (Schooten's relationship).
Theorem 3 (Fermat). T point has previously considered the minimum amount of property that makes MA + BT + MC M point of the plane triangle ABC.
Demonstration 1. Let M in the plane triangle ABC. At least one of quadrilaterals meningitis C, Master C, MAC B is convex. Be it MBA C. Applying Ptolemy's inequality, it follows: MA ≤ MB + MC so we get that: AA ≤ ≤ MA MA MA + + MB + MC.
Using Theorem 2 as follows: TA + TB + TC ≤ MA + MB + MC with equality if and only if M = T.
Deminstratia 2. (Using optical property of the ellipse).
Let M be the point of the plan for which the sum MA + MB + MC is minimal.
Prove that angles AMB, AMC, BMC 120 ° where v € arezulta using observation theorem, one that M = T.
Whether the absence of outbreaks B and C passing through M and A center circle and the radius AM.
The two curves are tangent at point M because otherwise, considering a point on the ellipse arc radius interiorulcercului AM, the sum of its distances from B and C is equal in value all the MB + MC (the definition of the ellipse) and distance from point A is lower than MA (point being situated inside the circle), in contradiction with the fact that M sum MA + MB + MC is minimal. Tnagenta Whether the two curves in M, d.
It follows that AM _ | _d, so I normal to M. The ellipse in optical property of the ellipse, angle MA is bisectuare for BMC, so AMBΞAMC. Analog is demonstrated that AMBΞBMC hence AMB, BMC, CMAΞ120 °, so M = T
Torricelli's 1.Teorema. Ferman's Theorem
Theorem 1 (Torricelli). Consider a triangle ABC in common with all angles strictly less than 120 ° SIP is being built in outer sides of the triangle equilateral triangles ABC, ACB, BCA. Circumscribed circles of these triangles have
Theorem 1 (Torricelli). Consider a triangle ABC in common.
Proof:
T be the point of intersection of the circles of triangles ABC and ACB. It proves that the quadrilaterals BTCA is writable. This is seen as quadrilaterals C BTA and TB were involved recordable BTAΞATC € 120 ° angles from which it follows that the quadrilateral BTCA is writable.
Note.
In this way, and demonstrated that there is a single point in the plane T with the property that ATB, ATC, BTC € 120 °, since T must belong to the three circles considered simultaneously. Point T is called Torricelli point for triunghil ABC's.
Theorem 2. Consider triangle ABC with all angles strictly less than 120 ° and equilateral triangles AB C, AC B BC up outside that in the previous theorem. Then:
a) AA, BB, CC are concurrent.
b) AT + BT + CT = DC = AA = BB
Proof. T be the point of competing circles of the problem before. It demonstrates that A, T, A are collinear, hence will result as AA, BB, CC are concurrent in T.
Uniting T to obtain: A TC, CBA, € € 60 ° and 120 ° ATC, ATA € 180 ° angle so.
For point b) proceed as follows: AA = AT + TA.
Ptolemy's theorem applies to recordable BTCA apentru quadrilateral (which is an equilateral triangle BCA) follows BT + TC = TA (Schooten's relationship).
Theorem 3 (Fermat). T point has previously considered the minimum amount of property that makes MA + BT + MC M point of the plane triangle ABC.
Demonstration 1. Let M in the plane triangle ABC. At least one of quadrilaterals meningitis C, Master C, MAC B is convex. Be it MBA C. Applying Ptolemy's inequality, it follows: MA ≤ MB + MC so we get that: AA ≤ ≤ MA MA MA + + MB + MC.
Using Theorem 2 as follows: TA + TB + TC ≤ MA + MB + MC with equality if and only if M = T.
Deminstratia 2. (Using optical property of the ellipse).
Let M be the point of the plan for which the sum MA + MB + MC is minimal.
Prove that angles AMB, AMC, BMC 120 ° where v € arezulta using observation theorem, one that M = T.
Whether the absence of outbreaks B and C passing through M and A center circle and the radius AM.
The two curves are tangent at point M because otherwise, considering a point on the ellipse arc radius interiorulcercului AM, the sum of its distances from B and C is equal in value all the MB + MC (the definition of the ellipse) and distance from point A is lower than MA (point being situated inside the circle), in contradiction with the fact that M sum MA + MB + MC is minimal. Tnagenta Whether the two curves in M, d.
It follows that AM _ | _d, so I normal to M. The ellipse in optical property of the ellipse, angle MA is bisectuare for BMC, so AMBΞAMC. Analog is demonstrated that AMBΞBMC hence AMB, BMC, CMAΞ120 °, so M = T